

Quantum vortex. In physics, a quantum vortex is a topological defect exhibited in superfluids and superconductors.
The existence of quantum vortices was predicted by Lars Onsager in 1947 in connection with superfluid helium. Onsager also pointed out that quantum vortices describe the circulation of superfluid and conjectured that their excitations are responsible for superfluid phase transitions. These ideas of Onsager were further developed by Richard Feynman in 1955 [1] and in 1957 were applied to describe the magnetic phase diagram of type-II superconductors by Alexei Alexeyevich Abrikosov[2] in the 1950s.
Quantum vortices are observed experimentally in Type-II superconductors, liquid helium, and atomic gases (see Bose–Einstein condensate). In a superfluid, a quantum vortex "carries" quantized angular momentum, thus allowing the superfluid to rotate; in a superconductor, the vortex carries quantized magnetic flux. Vortex in a superfluid[edit] where is Planck's constant divided by , we find along , then. Clausius–Clapeyron relation.
The Clausius–Clapeyron relation, named after Rudolf Clausius[1] and Benoît Paul Émile Clapeyron,[2] is a way of characterizing a discontinuous phase transition between two phases of matter of a single constituent.
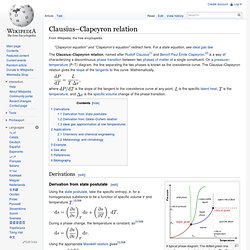
On a pressure–temperature (P–T) diagram, the line separating the two phases is known as the coexistence curve. The Clausius–Clapeyron relation gives the slope of the tangents to this curve. Mathematically, where is the slope of the tangent to the coexistence curve at any point, is the specific latent heat, is the temperature, and is the specific volume change of the phase transition.
Derivations[edit] A typical phase diagram. Derivation from state postulate[edit] Using the state postulate, take the specific entropy, , for a homogeneous substance to be a function of specific volume and temperature During a phase change, the temperature is constant, so[3]:508 Using the appropriate Maxwell relation gives[3]:508 Here and to the final phase Using the definition of specific enthalpy, , where .
M.K. Second sound. Second sound is a quantum mechanical phenomenon in which heat transfer occurs by wave-like motion, rather than by the more usual mechanism of diffusion.
Heat takes the place of pressure in normal sound waves. This leads to a very high thermal conductivity. It is known as "second sound" because the wave motion of heat is similar to the propagation of sound in air. Normal sound waves are fluctuations in the density of molecules in a substance; second sound waves are fluctuations in the density of phonons.[1] Second sound can be observed in any system in which most phonon-phonon collisions conserve momentum.
This occurs in superfluids,[2] and also in some dielectric crystals[3][4][5] when Umklapp scattering is small. Second sound in helium II[edit] Second sound is observed in liquid helium at temperatures below the lambda point, 2.1768 K, where 4He becomes a superfluid known as helium II. Second sound is also observed in superfluid helium-3 below its lambda point 2.5 mK.[11] References[edit] Superfluid. Superfluidity is a state of matter in which the matter behaves like a fluid with zero viscosity; where it appears to exhibit the ability to self-propel and travel in a way that defies the forces of gravity and surface tension.
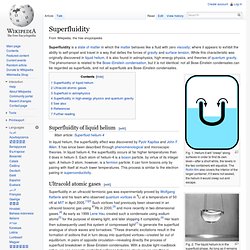
While this characteristic was originally discovered in liquid helium, it is also found in astrophysics, high-energy physics, and theories of quantum gravity. The phenomenon is related to the Bose–Einstein condensation, but it is not identical: not all Bose-Einstein condensates can be regarded as superfluids, and not all superfluids are Bose–Einstein condensates. Fig. 1. Helium II will "creep" along surfaces in order to find its own level—after a short while, the levels in the two containers will equalize.
The Rollin film also covers the interior of the larger container; if it were not sealed, the helium II would creep out and escape. Fig. 2. Superfluidity of liquid helium[edit] Ultracold atomic gases[edit] Superfluid in astrophysics[edit] See also[edit]