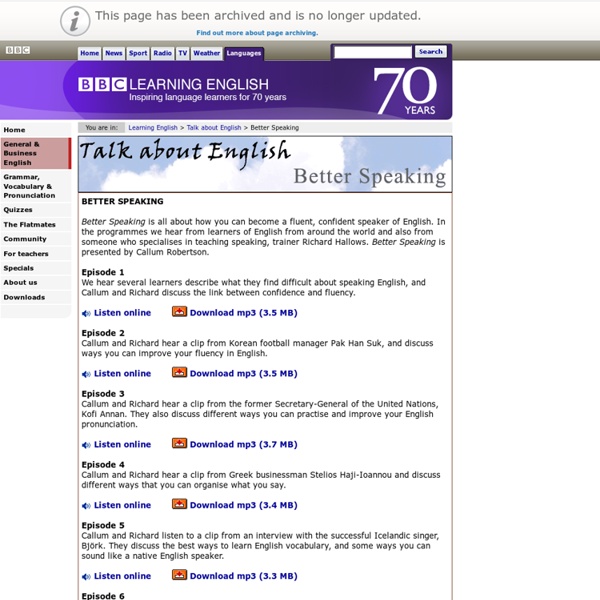
montyhall Un paradoxe probabiliste éclairé par les maths : le jeu des portes Ce paradoxe est décrit dans le film Las Vegas 21, la série NUMBERS (saison 1 - épisode 13), le livre Le Bizarre Incident du chien pendant la nuit de Mark Haddon... Aux Etats-Unis, de 1963 à 1977, le présentateur Monty Hall animait le jeu télévisé Let's Make a Deal.L'épreuve finale de ce jeu consistait à placer le candidat devant trois portes : derrière l'une d'entre elles il y avait une superbe voiture mais derrière les deux autres il y avait des chèvres. Le candidat choisissait une porte puis Monty Hall ouvrait alors une des deux portes restantes derrière laquelle il y avait une chèvre. Le candidat était alors libre de garder son choix de départ ou de le changer. Quelle était la meilleure stratégie ? Intuitivement on pourrait penser que ces deux stratégies sont équivalentes.Pourtant si vous effectuez plusieurs fois la simulation ci-dessous vous verrez que : Lien Wikipédia sur le problème de Monty Hall Etape n °1 Etape n °2
BBC Learning English | Talk about English - Private Lives Make Hyperbolic Tilings of Images The loaded image will be cropped to the centered hyperbolic polygon. Repeated hyperbolic reflections of the centered polygon make up the tiling. The option generate large generates a tiling that is larger than the image shown on the screen. The default scale factor for an enlarged tiling is four, this is a length factor. Another scale factor can be chosen below. Chrome may crash when trying to download a large image - "Aw, Snap!" Larger tilings take longer time to generate, mostly since the edge of the tiling gets finer. If change first tile is clicked, the rendering of the first tile will cycle through "no distortion", "Klein distortion", and "polynomial distortion". The hyperbolic tiling The tiling is made of regular hyperbolic polygons with sides. is the number of polygons meeting at each corner. When a so-called Poincaré disc is used to model hyperbolic geometry, the entire universe is inside a circle . Polynomial distortion Pick a point inside the Euclidean polygon. When then
tubequizard Enseigner les mathématiques comme DNL Sounds: The Pronunciation App [iPhone/iPad App] | Today's English in the World In this post I will discuss two apps by Macmillan Education (which I reviewed on my iPad): Macmillan Education. (2013a). Sounds: The Pronunciation App (Version 3.0) [Mobile application software]. Retrieved from Macmillan Education. (2013b). Cost: The free app is free, while the “premium” version costs $5.99 and has optional wordlists that can be purchased for $0.99 each. Menu for the free version of Sounds: The Pronunciation App Menu for the premium version of Sounds: The Pronunciation App Both of these apps are by Macmillan Education and appear to have been created with Adrian Underhill, who has an entire blog dedicated to the pronunciation chart featured in this app. Chart (both free and premium) Pronunciation Chart of American sounds in Sounds: The Pronunciation App The chart section in both versions presents the IPA symbols for the selected variety of English. Wordlist (only in the premium version) Sample definition for the word “duck” in Sounds: The Pronunciation App
Une carte interactive d'histoire des mathématiques Pour utiliser la carte en plein écran, cliquer sur l’icône en haut à droite L’approche historique et culturelle des mathématiques dans les programmes : de nouveaux angles pour l’apprentissage et l’enseignement de cette discipline Que ce soit dans les programmes de la refondation de l’école (2015) ou dans le référentiel de l’éducation prioritaire (2013), la question de la confrontation explicite des élèves aux dimensions culturelles et historiques des mathématiques est nouvelle. Toutefois, depuis de nombreuses années, on trouve des travaux de recherche [1] et de nombreux ouvrages pédagogiques [2] et culturels qui irriguent ces formes d’enseignements, celles-ci étant plus ou moins mises en œuvre. Dorénavant, suite à la refondation de l’École, le socle commun a été enrichi du terme « culture », venant s’ajouter à ceux de « connaissances » et « compétences » déjà existants. Un faisceau de nouvelles questions se présente à l’enseignant : L. G. Comment utiliser la carte ?
Six Amazing Websites that Make Your Writing Stronger Long writing activities are not very frequently done in class. I tend to think that my students are like me; I need the right kind of atmosphere. Writing requires time, silence and lots of inspiration. Ideally, at this time of the year, I would probably wish to be sitting next to a fireplace with the most perfect instagrammable snow falling outside my window while drinking a nice cup of coffee waiting for inspiration to strike. Unfortunately, there isn’t any snow where I live so I’ll have to make do with a bit of rain and some reddish trees. Note: you won’t find “instagrammable” in the dictionary Inspiration, the most important word when writing and something my students claim to lack. These are some great sites that can help you make your writing stronger. Photo by Tekke 1. Skell is easy to use. 2.Netspeak is a really helpful site to help you write better. You can find the word(s) you’re looking for by typing signs as seen in the picture below. Type ? 3. 4. 5. 6.
Mathematics Introduce your students to the wonderful world of mathematics with our collection of lessons for young learners. Each worksheet comes with a set of clear and comprehensive teacher's notes providing guidance and ideas for extensions activities. Help your pupils learn key mathematical concepts by applying them to their own daily activities with this selection of fun and engaging worksheets. Matching, drawing and colouring activities make learning about maths in English fun. Counting with coinsPupils will have been working on identifying and using rhyming words in their own language: in these activities learners complete poems and play a memory game using rhyming words in English.
Books - Words To Use action adventure audiobook autobiography biography children's christian classics comedy comic cook book crime cult classic drama fantasy fiction foreign health history hobby book horror independent indie kids' memoir mystery non-fiction photography reference romance sci-fi science fiction self-help short story special interest suspense technical teens textbook thriller travel war drama Western
Kangaroo Maths - Kenny's Pouch Resources based on the rich history of mathematics and its place in different cultures Characters & Concepts | Codes & Ciphers | National Lottery | Mathemagic | Pascal's Triangle | Fibonacci Numbers | National Lottery | Mathematician of the Month | Maths Trail Mathematical Characters and Concepts Codes and Ciphers - Frequency Analysis First, a series of posters on the History of Codes. You will need these two graphs to make any sense of them. Codes and Ciphers - Everyday Codes The Maths behind barcodes and ISBN's is revealed through this series aimed at Year 8. Codes and Ciphers - Scrambling and Hiding The first in a series of themed lessons (this is aimed at Year 7). The National Lottery Extensive teacher notes for a possible project. Mathemagic Teacher notes for a possible project. Pascal's Triangle A mostly blank template to be filled in.The first 12 rows, with rows and diagonals numbered.The first 50 rows displayed on a spreadsheet. Fibonacci Numbers and the Golden Ratio Some alternatives!
factitious Listening Comprehension, Listening that sparks learning | Listenwise Cours d'anglais gratuit | Apprendre l'anglais Comment apprendre l'anglais seul ? Commencez par un cours facile et gratuit sur Internet ! Nous avons adopté une démarche pratique et concrète pour apprendre à parler une langue facilement et rapidement : nous vous proposons de commencer par mémoriser des mots, des phrases et des expressions pratiques que vous pourrez utiliser tous les jours chez vous pour vous entraîner et qui vous seront ensuite utiles en voyage. S'entraîner à prononcer à voix haute du vocabulaire, les nombres par exemple, est un bon exercice qui peut se pratiquer à tout moment de la journée. Pourquoi parler l'anglais en voyage ? Pour échanger avec le monde entier Parler cette langue, même s'il ne s'agit que de quelques mots et expressions, c'est s'assurer de pouvoir échanger avec la plupart des habitants du monde durant ses vacances à l'étranger. Un marqueur social et professionnel Réussir votre séjour dans un pays anglophone La deuxième langue la plus parlée au monde L'alphabet anglais → BBC Learning English