

Tool Use in an Innovative Learning Arrangement for Mathematics. Ulrich C., Tillema E. S., Hackenberg A. J. & Norton A. (2014) Constructivist Model Building: Empirical Examples From Mathematics Education. Constructivist Foundations 9(3): 328–339. 4.2.1 Making Triangles. Task Using one band for each triangle, make as many different sizes and shapes of triangles, as you can on the computer geoboard.
Explain to a friend the ways in which these triangles are different and how they are alike. [Stand-alone applet] How to Use the Interactive Figure We will refer to the pegs that the rubber band is attached to as nodes. To attach the rubber band to more than two nodes, drag the rubber band from the middle.To move a rubber band to a new node, click on the current node and drag the rubber band to the desired node.To remove a rubber band from a node, click on the node to select it (a double circle appears), then click on the Delete Node button.To delete a rubber band, click on it to select it, then click the Delete Band button.To clear the geoboard, click on the Clear All button.To color the interior of a shape, click on the rubber band and then click on a color.
Students enjoy working with geoboards, whether they are interactive computer geoboards or physical ones. 4.2 Investigating Geometry Concepts on GeoBoards. Becoming a Teacher Through Action Research, 2nd Edition - Resources. Evaluating questionnaires with cognitive testing - NCRM EPrints Repository. Making Mathematics: Support for Teachers. "I can think of two good criteria ... for deciding what to teach: whether the knowledge gives a sense of delight and whether it bestows the gift of intellectual travel beyond the information given, in the sense of containing within it the basis of generalization.
" —Jerome Bruner Mathematics is a discipline with the potential for important insights, beautiful symmetries, and unexpected discoveries. All of our students are capable of experiencing this potential for themselves. From 1999-2002, the Making Mathematics project provided mathematicians as mentors and curricular materials to help teachers and students explore the full richness of our discipline together. Selected Papers 1996. Intersubjectivity in Mathematics Learning: A Challenge to. Intersubjectivity in Mathematics Learning: A Challenge to the Radical Constructivist Paradigm?

This paper appeared in "Journal for Research in Mathematics Education" Vol. 27, No. 2, p. 133-150, March 1996 Abstract Radical constructivism is currently a major, if not the dominant, theoretical orientation in the mathematics education community, in relation to children's learning. There are, however, aspects of children's learning which are challenges to this perspective, and what appear to be "at least temporary states of intersubjectivity" (Cobb, Wood & Yackel 1991 p. 162) in the classroom is one such challenge.
In this paper I discuss intersubjectivity, and through it offer an examination of the limitations of the radical constructivist perspective. Constructivists, whether radical, weak or social (Cobb 1994), draw their inspiration from Piaget for whom the individual is the central element in meaning-making. Intersubjectivity and Social Constructivism More poetically, Vygotsky says (1986): Paradigms. Learning theories tend to fall into one of several perspectives or paradigms, including behaviorism, cognitivism, constructivism, and others.
Here are some of the basic ones: Behaviorism Founders and proponents: John B. Watson in the early 20th century. English - Leerplanevaluatie. Formative evaluation Curriculum developers use a cyclical approach to increase the quality of the product.
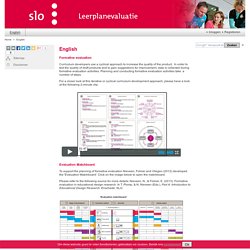
In order to test the quality of draft products and to gain suggestions for improvement, data is collected during formative evaluation activities. Planning and conducting formative evaluation activities take a number of steps. For a closer look at this iterative or cyclical curriculum development approach, please have a look at the following 2-minute clip: Evaluation Matchboard To support the planning of formative evaluation Nieveen, Folmer and Vliegen (2012) developed the 'Evaluation Matchboard'.
Please refer to the following source for more details: Nieveen, N., & Folmer, E. (2013). Reference Materials. Creating a Mathematics Classroom Environment. Improving Mathematical Problem Solving in Grades 4 Through 8: What Works Clearinghouse. Perasma apo tin arithmitiki stin algebra.pdf. Encouraging Mathematical Thinking: Introduction. Being a professional educator takes time -- time to plan, time to practice, time to grade, time to communicate -- and I never have enough time.

However, I now realize that adding reflection and research to my agenda have made my life as a teacher easier, not more difficult. -- Judith Koenig, Project teacher This paper reflects our working knowledge about how teachers engage students in mathematical thinking, and why this is important. We focus in particular on discourse in the classroom. 53 - Active Learning Strategies in Face-to-Face Courses. Longitudinal proof in mathematics project - introduction. ConstructMap - BEARWiki. Finding Pi by Archimedes' Method. Research Sampler 8: Students' difficulties with proof. By Keith Weber What is proof and what is its role in mathematics?

What difficulties do students have with proofs? How can the concept of proof be taught effectively? References Proof is a notoriously difficult mathematical concept for students. What is proof and what is its role in mathematics? Many mathematicians and mathematics teachers would consider the answers to this question straightforward. Fields Medalist William Thurston [1994] argues that it is important to distinguish between formal proofs and proofs that mathematicians actually construct. Davis and Hersh [1981] argue that it is probably impossible to define precisely what type of argument will be accepted as a valid proof by the mathematical community. Paul Ernest Paper. Paul Ernest University of Exeter Epistemological issues, although controversial, are central to teaching and learning and have long been a theme of PME.

A central epistemological issue is that of the philosophy of mathematics. Understanding and interpreting box plots. How to read a box plot/Introduction to box plots Box plots are drawn for groups of W@S scale scores.

They enable us to study the distributional characteristics of a group of scores as well as the level of the scores.