

Sacred geometry. As worldview and cosmology[edit] The belief that God created the universe according to a geometric plan has ancient origins.
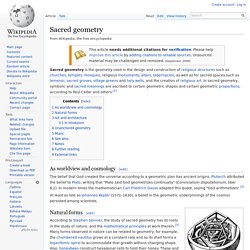
Plutarch attributed the belief to Plato, writing that "Plato said God geometrizes continually" (Convivialium disputationum, liber 8,2). In modern times the mathematician Carl Friedrich Gauss adapted this quote, saying "God arithmetizes".[2] At least as late as Johannes Kepler (1571–1630), a belief in the geometric underpinnings of the cosmos persisted among scientists. Closeup of inner section of the Kepler's Platonic solid model of planetary spacing in the Solar system from Mysterium Cosmographicum (1596) which ultimately proved to be inaccurate Natural forms[edit] Art and architecture[edit] Geometric ratios, and geometric figures were often employed in the design of Egyptian, ancient Indian, Greek and Roman architecture.
In Hinduism[edit] Unanchored geometry[edit] Music[edit] See also[edit] Notes[edit] Further reading[edit] External links[edit] Sacred geometry at DMOZ. The Theory of Abstract Objects. Home Page Introduction.

The laws list. Erasing history? Temporal cloaks adjust light's throttle to hide an event in time. Researchers from Cornell University in Ithaca, N.Y., have demonstrated for the first time that it's possible to cloak a singular event in time, creating what has been described as a "history editor.
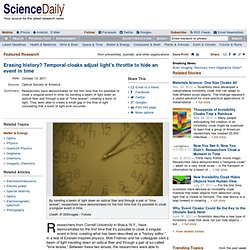
" In a feat of Einstein-inspired physics, Moti Fridman and his colleagues sent a beam of light traveling down an optical fiber and through a pair of so-called "time lenses. " Between these two lenses, the researchers were able to briefly create a small bubble, or gap, in the flow of light. During that fleetingly brief moment, lasting only the tiniest fraction of a second, the gap functioned like a temporal hole, concealing the fact that a brief burst of light ever occurred. The team is presenting their findings at the Optical Society's (OSA) Annual Meeting, Frontiers in Optics (FiO) 2011 ( taking place in San Jose, Calif. next week. Lectures. Public lectures given at the Institute for Advanced Study. Lectures. Free university lectures - computer science, mathematics, physic.
Whether your goal is to earn a promotion, graduate at the top of your class, or just accelerate your life, lectures can help get you there.

Our archives of lectures cover a huge range of topics and have all been handpicked and carefully designed by experienced instructors throughout the world who are dedicated to helping you take the next step toward meeting your career goals. Lifelong learns can turn their free time turn into self-improvement time. The online lectures on this list are more than lecture notes or a slideshow on a topic -- they were designed for audiences like you, with carefully sequenced themes and topics taught by veteran educators, and often with additional resources for your own independent study.
College Physics. Physics text - Contents. Einstein for Everyone - StumbleUpon. Einstein for Everyone.

MathAppendices. Elementary Concepts in Statistics. In this introduction, we will briefly discuss those elementary statistical concepts that provide the necessary foundations for more specialized expertise in any area of statistical data analysis.

The selected topics illustrate the basic assumptions of most statistical methods and/or have been demonstrated in research to be necessary components of our general understanding of the "quantitative nature" of reality (Nisbett, et al., 1987). We will focus mostly on the functional aspects of the concepts discussed and the presentation will be very short. Further information on each of the concepts can be found in statistical textbooks.
Recommended introductory textbooks are: Kachigan (1986), and Runyon and Haber (1976); for a more advanced discussion of elementary theory and assumptions of statistics, see the classic books by Hays (1988), and Kendall and Stuart (1979). What are Variables? Variables are things that we measure, control, or manipulate in research. Correlational vs. Dependent vs. Calculus Video Tutorials. Discrete Math Lecture Notes. Pauls Online Math Notes. Russell's Paradox. First published Fri Dec 8, 1995; substantive revision Tue Dec 3, 2013 Russell's paradox is the most famous of the logical or set-theoretical paradoxes.
Also known as the Russell-Zermelo paradox, the paradox arises within naïve set theory by considering the set of all sets that are not members of themselves. Such a set appears to be a member of itself if and only if it is not a member of itself. Hence the paradox. Some sets, such as the set of all teacups, are not members of themselves. Although also noticed by Ernst Zermelo, the contradiction was not thought to be important until it was discovered independently by Bertrand Russell in the spring of 1901. 1. Is R a member of itself? As Russell tells us, it was after he applied the same kind of reasoning he found in Cantor's diagonal argument to a “supposed class of all imaginable objects” that he was led to the contradiction: 2. Russell wrote to Frege with news of his paradox on June 16, 1902. 3.
As Whitehead and Russell explain, 4. Quadratic Equations: Quadratic Formula. Consider the general quadratic equation with .

The Most IMPORTANT Video You'll Ever See - FULL LECTURE HD.
Read biographies of Famous Philosophers, famous Philosopher biography, world famous Philosophers, Great Indian Philosophers - Incredible-people.com.