

Deductive reasoning. Deductive reasoning links premises with conclusions.
Abductive reasoning. Abductive reasoning (also called abduction,[1] abductive inference[2] or retroduction[3]) is a form of logical inference that goes from an observation to a hypothesis that accounts for the observation, ideally seeking to find the simplest and most likely explanation.
In abductive reasoning, unlike in deductive reasoning, the premises do not guarantee the conclusion. Raven paradox. The raven paradox suggests that both of these images contribute evidence to the supposition that all ravens are black.
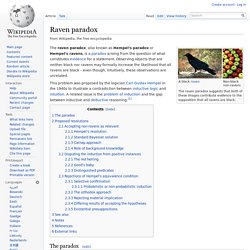
The raven paradox, also known as Hempel's paradox or Hempel's ravens, is a paradox arising from the question of what constitutes evidence for a statement. Observing objects that are neither black nor ravens may formally increase the likelihood that all ravens are black – even though, intuitively, these observations are unrelated. Inductive reasoning. Inductive reasoning (as opposed to deductive reasoning or abductive reasoning) is reasoning in which the premises seek to supply strong evidence for (not absolute proof of) the truth of the conclusion.
While the conclusion of a deductive argument is certain, the truth of the conclusion of an inductive argument is probable, based upon the evidence given.[1] The philosophical definition of inductive reasoning is more nuanced than simple progression from particular/individual instances to broader generalizations. Rather, the premises of an inductive logical argument indicate some degree of support (inductive probability) for the conclusion but do not entail it; that is, they suggest truth but do not ensure it. In this manner, there is the possibility of moving from general statements to individual instances (for example, statistical syllogisms, discussed below).
Mill's Methods. Mill's Methods are five methods of induction described by philosopher John Stuart Mill in his 1843 book A System of Logic.[1] They are intended to illuminate issues of causation.
The methods[edit] Direct method of agreement[edit] If two or more instances of the phenomenon under investigation have only one circumstance in common, the circumstance in which alone all the instances agree, is the cause (or effect) of the given phenomenon. For a property to be a necessary condition it must always be present if the effect is present. Since this is so, then we are interested in looking at cases where the effect is present and taking note of which properties, among those considered to be 'possible necessary conditions' are present and which are absent.
A B C D occur together with w x y z A E F G occur together with w t u v. Inductive reasoning. Deductive reasoning. Mathematical proof. One of the oldest surviving fragments of Euclid's Elements, a textbook used for millennia to teach proof-writing techniques.
The diagram accompanies Book II, Proposition 5.[1] In mathematics, a proof is a deductive argument for a mathematical statement. In the argument, other previously established statements, such as theorems, can be used. In principle, a proof can be traced back to self-evident or assumed statements, known as axioms.[2][3][4] Proofs are examples of deductive reasoning and are distinguished from inductive or empirical arguments; a proof must demonstrate that a statement is always true (occasionally by listing all possible cases and showing that it holds in each), rather than enumerate many confirmatory cases.
An unproved proposition that is believed true is known as a conjecture. Proofs employ logic but usually include some amount of natural language which usually admits some ambiguity. History and etymology[edit] Nature and purpose[edit] Methods[edit] Direct proof[edit] . Formal proof. The theorem is a syntactic consequence of all the well-formed formulas preceding it in the proof.
For a well-formed formula to qualify as part of a proof, it must be the result of applying a rule of the deductive apparatus of some formal system to the previous well-formed formulae in the proof sequence. Formal proofs often are constructed with the help of computers in interactive theorem proving. Significantly, these proofs can be checked automatically, also by computer. Checking formal proofs is usually simple, while the problem of finding proofs (automated theorem proving) is usually computationally intractable and/or only semi-decidable, depending upon the formal system in use.
Background[edit] Philosophic burden of proof. Not Sentience. Philosophy and sentience[edit] In the philosophy of consciousness, sentience can refer to the ability of any entity to have subjective perceptual experiences, or as some philosophers refer to them, "qualia".[2] This is distinct from other aspects of the mind and consciousness, such as creativity, intelligence, sapience, self-awareness, and intentionality (the ability to have thoughts "about" something).
Sentience is a minimalistic way of defining consciousness, which is otherwise commonly used to collectively describe sentience plus other characteristics of the mind. Some philosophers, notably Colin McGinn, believe that sentience will never be understood, a position known as "new mysterianism". They do not deny that most other aspects of consciousness are subject to scientific investigation but they argue that subjective experiences will never be explained; i.e., sentience is the only aspect of consciousness that can't be explained.
Necessary and Sufficient Conditions. 1.
Philosophy and Conditions An ambition of twentieth-century philosophy was to analyse and refine the definitions of significant terms—and the concepts expressed by them—in the hope of casting light on the tricky problems of, for example, truth, morality, knowledge and existence that lay beyond the reach of scientific resolution. Central to this goal was specifying at least in part the conditions to be met for correct application of terms, or under which certain phenomena could truly be said to be present. Reason. Psychologists and cognitive scientists have attempted to study and explain how people reason, e.g. which cognitive and neural processes are engaged, and how cultural factors affect the inferences that people draw.
The field of automated reasoning studies how reasoning may or may not be modeled computationally. Animal psychology considers the question of whether animals other than humans can reason. Etymology and related words[edit] In the English language and other modern European languages, "reason", and related words, represent words which have always been used to translate Latin and classical Greek terms in the sense of their philosophical usage. The original Greek term was "λόγος" logos, the root of the modern English word "logic" but also a word which could mean for example "speech" or "explanation" or an "account" (of money handled).[7]As a philosophical term logos was translated in its non-linguistic senses in Latin as ratio. Digital Reasoning. Inductive Reasoning - How Small Observations Infers a Theory. Inductive reasoning is the process where a small observation is used to infer a larger theory, without necessarily proving it.
Most scientists use this method to generate theories about how the universe works and discover the laws governing our very existence. Many ancient philosophers used induction for making observations and constructing theories. For example, the Ancient Greek philosophers believed that theories could be proved by logic alone and did not need experiments. They thought that mathematically strict laws, deduced from smaller observations, governed the universe. Science has moved on over the millennia and now we realize the necessity of research by deductive reasoning. Theories have to be tested and hypotheses answered before the scientific community accepts them as truth. Generally speaking, inductive reasoning and deductive reasoning are a circular process and inextricably linked. Deductive and inductive reasoning.