

Cómo mejorar a las abejas con fractales. Este texto es una colaboración del Cuaderno de Cultura Científica con Next Las estructuras en forma de hexágono de los paneles de abeja, como las que se encuentran en las colmenas, son conocidas, entre otras cosas, porque presentan unas características mecánicas notables, como una rigidez extremadamente alta en relación a su densidad.
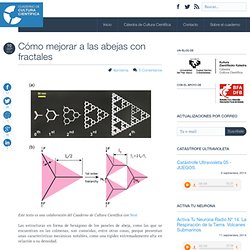
Ahora un grupo de investigadores, encabezados por Ramin Oftadeh, de la Northeastern University (EE.UU.), demuestran que las propiedades mecánicas de estos paneles uniformes pueden ser aún mejores si se añaden distintos niveles jerárquicos para terminar formando materiales fractales. Estos resultados, que se publican en Physical Review Letters, proporcionan una ruta fácil para mejorar y afinar el rendimiento de materiales cambiando detalles de su estructura hexagonal, algo que cada vez es más fácil de conseguir con el uso de impresoras 3D.
Los investigadores modularon la densidad del conjunto simplemente alterando el grosor de las paredes de los hexágonos. 21 GIFs That Explain Mathematical Concepts. “Let's face it; by and large math is not easy, but that's what makes it so rewarding when you conquer a problem, and reach new heights of understanding.”
Danica McKellar As we usher in the start of a new school year, it’s time to hit the ground running in your classes! Math can be pretty tough, but since it is the language in which scientists interpret the Universe, there’s really no getting around learning it. Check out these gifs that will help you visualize some tricky aspects of math, so you can dominate your exams this year.
Ellipse: Via: giphy Solving Pascal triangles: Via: Hersfold via Wikimedia Commons. Online LaTeX Equation Editor - create, integrate and download. This Mathematical Equation Predicts Momentary Happiness. What is the secret to achieving happiness?
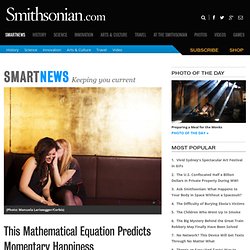
According to some experts, it is the following formula: As the BBC reports, researchers from the University College London say that that formula closely predicts momentary bouts of happiness. The equation is built around the relationship between risk-taking, past experiences and reward. Just as a surprisingly tasty meal at a new restaurant can bring pleasure, the researchers explained to the BBC, so, too, can looking forward to a dinner at a favorite spot. The equation, they say, balances that nuance. To design it, the researchers first asked 26 participants to play a simple game that involved risk-taking and potential rewards and to report how happy they were after each round, the BBC reports.
After devising the formula, the team recruited around 18,000 people to play a risk-reward game on their smartphones. "It is often said that you will be happier if your expectations are lower," they told SBS. John Venn Google doodle: the right way to do a Venn diagram. Hull-born John Venn, creator of the eponymous diagram, was born 180 years ago today and to celebrate Google have created an interactive doodle of the famous data visualisation.
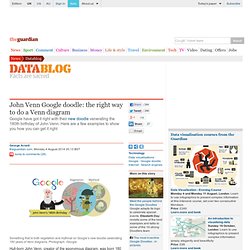
The concept of the Venn is simple. In each section (in most cases represented as a circle) you have one limited collection of things. Mathematical equation to predict happiness: Doesn't depend on how well things go, but on whether things are better than expected. Mathematical Masterpieces: Making Art From Equations. Artist Annie Verhoeven has developed a very unusual technique to make her artwork, which she has termed “thread art.” Math-Inspired Works of Art. “It works because the floating tetrahedra want to fall apart into five pieces and the floating pentagons want to fall apart into six pieces,” Hart says.
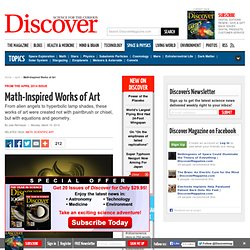
“They can’t agree on how to fall apart, so they end up staying together.” Each of the two smaller structures has 30 sides: six pentagons with five sides each, and five tetrahedra with six edges each. Each pentagon side connects with a single tetrahedron edge — this is what gives the sculpture its solidity. Hart chose sharply waved edges for the pentagons and allowed the edges of his tetrahedra to stray from straight lines, undulating first up and then down in a wave.
The sculpture is thus created from 30 laser-cut wood pieces, each containing one pentagon edge and one tetrahedron edge. Math explains history: Simulation accurately captures the evolution of ancient complex societies. The question of how human societies evolve from small groups to the huge, anonymous and complex societies of today has been answered mathematically, accurately matching the historical record on the emergence of complex states in the ancient world.
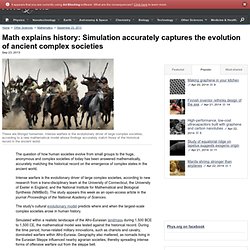
Intense warfare is the evolutionary driver of large complex societies, according to new research from a trans-disciplinary team at the University of Connecticut, the University of Exeter in England, and the National Institute for Mathematical and Biological Synthesis (NIMBioS). The study appears this week as an open-access article in the journal Proceedings of the National Academy of Sciences. The study's cultural evolutionary model predicts where and when the largest-scale complex societies arose in human history. In Love with Geometry. In this animation by Keenan Crane, a surface in the shape of a bunny flows to a sphere.
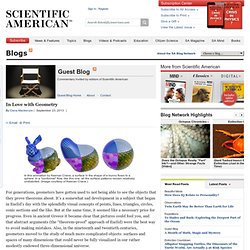
In a "conformal" flow, like this one, all the surface patterns remain relatively undistorted. (Image courtesy of Keenan Crane.) For generations, geometers have gotten used to not being able to see the objects that they prove theorems about. Project Euler.