

Weyl's lemma (Laplace equation) In mathematics, Weyl's lemma, named after Hermann Weyl, states that every weak solution of Laplace's equation is a smooth solution.
This contrasts with the wave equation, for example, which has weak solutions that are not smooth solutions. Weyl's lemma is a special case of elliptic or hypoelliptic regularity. Let be an open subset of -dimensional Euclidean space , and let denote the usual Laplace operator. Weakly harmonic function. Schwarz reflection principle. This article is about the reflection principle in complex analysis.
For reflection principles of set theory, see Reflection principle. Edmund Schuster. Edmund Schuster (7 September 1851 – 5 July 1932) was a German engineer and mathematician who contributed to the field of special functions and complex analysis being a pioneer in the field of harmonic analysis.

Schuster was born in Munich in 1851. He studied in Bonn and Leipzig, and in 1875 he moved with his family to Chile. Poisson kernel. In potential theory, the Poisson kernel is an integral kernel, used for solving the two-dimensional Laplace equation, given Dirichlet boundary conditions on the unit disc.
The kernel can be understood as the derivative of the Green's function for the Laplace equation. It is named for Siméon Poisson. Poisson kernels commonly find applications in control theory and two-dimensional problems in electrostatics. In practice, the definition of Poisson kernels are often extended to n-dimensional problems. Two-dimensional Poisson kernels[edit] Pluriharmonic function. Formal definition[edit] formed by using every couple of complex tuples a, b ∈ ℂn, the function is a harmonic function on the set Basic properties[edit] Every pluriharmonic function is a harmonic function, but not the other way around.
Further, it can be shown that for holomorphic functions of several complex variables the real (and the imaginary) parts are locally pluriharmonic functions. See also[edit] Notes[edit] Historical references[edit] References[edit] Amoroso, Luigi (1912), "Sopra un problema al contorno", Rendiconti del Circolo Matematico di Palermo (in Italian) 33 (1): 75–85, doi:10.1007/BF03015289, JFM 43.0453.03 . Newtonian potential. The Newtonian potential of a compactly supported integrable function ƒ is defined as the convolution where the Newtonian kernel Γ in dimension d is defined by Here ωd is the volume of the unit d-ball, and sometimes sign conventions may vary; compare (Evans 1998) and (Gilbarg & Trudinger 1983).
Maximum principle. This article describes the maximum principle in the theory of partial differential equations.

For the maximum principle in optimal control theory, see Pontryagin's minimum principle. Laplace's equation. In mathematics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace who first studied its properties.
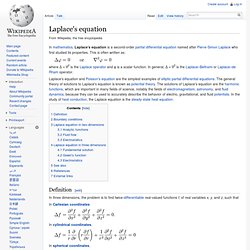
This is often written as: Laplace operator. The Laplace operator is named after the French mathematician Pierre-Simon de Laplace (1749–1827), who first applied the operator to the study of celestial mechanics, where the operator gives a constant multiple of the mass density when it is applied to a given gravitational potential.
Solutions of the equation ∆f = 0, now called Laplace's equation, are the so-called harmonic functions, and represent the possible gravitational fields in free space. The Laplacian occurs in differential equations that describe many physical phenomena, such as electric and gravitational potentials, the diffusion equation for heat and fluid flow, wave propagation, and quantum mechanics. Kellogg's theorem. Hilbert transform. The Hilbert transform, in red, of a square wave, in blue Introduction[edit] The Hilbert transform of u can be thought of as the convolution of u(t) with the function h(t) = 1/(πt).

Because h(t) is not integrable, the integrals defining the convolution do not converge. Instead, the Hilbert transform is defined using the Cauchy principal value (denoted here by p.v.). Harnack's principle. Harnack's inequality. The statement[edit] A harmonic function (green) over a disk (blue) is bounded from above by a function (red) that coincides with the harmonic function at the disk center and approaches infinity towards the disk boundary.
Harnack's inequality applies to a non-negative function f defined on a closed ball in Rn with radius R and centre x0. It states that, if f is continuous on the closed ball and harmonic on its interior, then for any point x with |x - x0| = r < R. Harmonic coordinates. Harmonic conjugate. In mathematics, a function defined on some open domain is said to have as a conjugate a function if and only if they are respectively real and imaginary part of a holomorphic function. Dirichlet's principle. Cauchy–Riemann equations. In the field of complex analysis in mathematics, the Cauchy–Riemann equations, named after Augustin Cauchy and Bernhard Riemann, consist of a system of two partial differential equations which, together with certain continuity and differentiability criteria, form a necessary and sufficient condition for a complex function to be complex differentiable, that is holomorphic.
This system of equations first appeared in the work of Jean le Rond d'Alembert (d'Alembert 1752). Later, Leonhard Euler connected this system to the analytic functions (Euler 1797). Cauchy (1814) then used these equations to construct his theory of functions. Harmonic function. This article is about harmonic functions in mathematics. For harmonic function in music, see diatonic functionality. everywhere on U. This is usually written as or Examples[edit] Examples of harmonic functions of two variables are: