

MATHEMATICAL SEMIOTICS. Semiotics in diamonds-verdana.nb. Rudolf Kaehr Dr.@ ThinkArt Lab Glasgow Abstract Semiotics are embedded into diamonds in a double way. 1. 1.1.

Introduction of the semiotic scheme by Bense/Toth: An extensive study of a relational introduction of the Peircean semiotics is presented in [Toth, Einführung in die semiotische Relationentheorie]. 1.1.1. A diamondization of the semiotic scheme is naturally realized as a diamond interpretation of the semiotic relations, i.e. morphisms. Firstness as a doubletA composition always is accompanied by an environment of its morphisms. Comments1Therefore,Firstness is a relation to itself, a ‘monadic’ relation:a as a a is a reduction of a a a.Secondness is a binary or ‘dyadic’ relation:a b is a reduction of a b b, a b.Thirdness is a ternary or ‘triadic’ relation:a c is a realization of the intuition.
Classificatory remarks2 In Transit (Toth, 2008)3 For a start, it might be a significant result to proof the difference with the simple example of Toth’ s dually invariant sign class 1.2. 1.2.1. 2. Isotopy (semiotics) The concept was highly influential and has been revisited and redefined by multiple authors, starting from Greimas, to his epigons of the Paris school, Umberto Eco,[5] the Groupe µ, and others.
Greimas initial definition was based on the concept of repetition (also termed recurrence or redundancy), was focused on semantics as it only regarded the repetition semes, and it stressed the role of isotopy of making possible a uniform reading of a story and resolving ambiguities. To quote his first 1966 formulation: "a redundant set of semantic categories which make possible the uniform reading of the story. "[6][7][8] In 1980 Umberto Eco showed a flaw of using the concept of "repetition". Catherine Kerbrat-Orecchioni systematically extended the concept of isotopy to denote the repetition of any semiotic unit. A semantic isotopy, the narrower and most common type of isotopy, the one of Greimas's original definition, is the recurrence of semes. Semiotic square. Basic structure[edit] The Greimas Square is a model based on relationships: The Semiotic Square is formed by an initial binary relationship between two contrary signs.
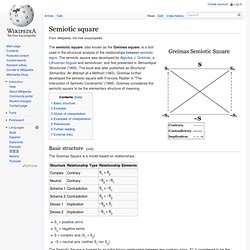
S1 is considered to be the assertion/positive element and S2 is the negation/negative element in the binary pair: The second binary relationship is now created on the ~S axis. ~S1 is considered to be the complex term, and ~S2 is the neutral term. In most modes of interpretation, the S-axis is a hyponym of the ~S-axis. Finally, the ~S2 element can be identified. Example[edit] The Semiotic square - also known as Greimas' rectangle or semantic rectangle - is a way of classifying concepts which are relevant to a given opposition of concepts, such as feminine-masculine, beautiful-ugly, etc. and of extending the relevant ontology. Starting from a given opposition of concepts S1 and S2, the semiotic square entails first the existence of two other concepts, namely ~S1 and ~S2, which are in the following relationships:
Algebraic Semiotics. Algebraic Semiotics Contents Short Overview Algebraic semiotics is a new approach to meaning and representation, and in particular to user interface design, that builds on five important insights from the last hundred years: Semiotics: Signs are not isolated items; they come in systems, and the structure of a sign is to a great extent inherited from the system to which it belongs.

Signs do not have pre-given "Platonic" meanings, but rather their meaning is relational, because signs are always interpreted in particular contexts. A basic concept is that of a semiotic morphism, which provides representations in one sign system (the target) for signs from another (the source). To illustrate these ideas, consider proofs (in some fixed logical system) as forming a sign system; an extension of this system includes additional information to help with understanding proofs, such as motivation, background tutorials, and examples.
Some Concrete Applications Social Foundations.